A recent question reminded me I hadn’t yet written about the complexity surrounding the definition of ratio (and related terms, like rate and fraction). Here are four questions about the words.
Are rates ratios? Are ratios rates?
We’ll start with this 2001 question about two definitions:
Rate vs. Ratio In a class with other math teachers, we were discussing the relation of rate versus ratio. Are all rates ratios and are all ratios rates? What is your take on this and how would you explain this?
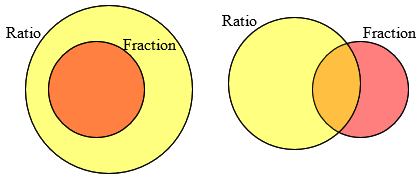
That is, would a Venn diagram look like this (all rates are ratios):
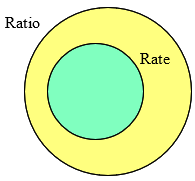
Or like this (all ratios are rates):
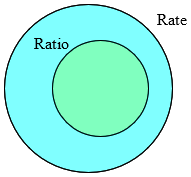
or something else?
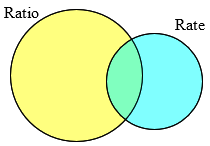
I answered with some research (some links have been redirected to Archive.org):
Hi, Stacy. I've seen different ways to distinguish these concepts, so I looked around to find some samples. This page Math League, Ratio and Proportion http://www.mathleague.com/help/ratio/ratio.htm says "A ratio is a comparison of two numbers. "A rate is a ratio that expresses how long it takes to do something, such as traveling a certain distance. To walk 3 kilometers in one hour is to walk at the rate of 3 km/h. The fraction expressing a rate has units of distance in the numerator and units of time in the denominator." This approach is more or less what I use in real life; ratio is the general concept, and rate refers _usually_ to time (though not always, as I'll mention below). So all rates are ratios.
That is, “rate” is a specific kind of ratio; but this makes it a little too specific.
But it's common to make a different distinction: Jim Reed, Rate and Ratio http://dev1.epsb.edmonton.ab.ca/math14_Jim/math7/strand1/1208.htm (which, ironically, has a link to the page above) says "Rate: A comparison of 2 measurements with different units." "Ratio: A comparison of numbers with the same units so units are not required." This makes ratio and rate both specific terms, so that neither is a special case of the other. That seems very orderly, but I don't like it, because (a) generic terms are very useful, in order to be able to talk about how we work with ANY entity of this sort, and (b) this doesn't fit common usage, as in "tax rate" or "interest rate," where both numbers have the same unit. Of course, math doesn't generally worry too much about common usage, but since I see no real mathematical advantage in this distinction, we might as well be realistic.
This would make them distinct concepts:
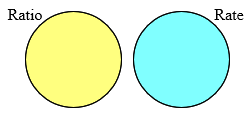
But inclusive definitions are generally preferred in math. We need a word that covers both!
The next, MathSteps, Rates http://www.eduplace.com/math/mathsteps/6/e/ says "A ratio is a comparison of two numbers or measurements. The numbers or measurements being compared are called the terms of the ratio. A rate is a special ratio in which the two terms are in different units. For example, if a 12-ounce can of corn costs 69 cents, the rate is 69 cents for 12 ounces. The first term of the ratio is measured in cents; the second term in ounces." This makes ratio generic again, satisfying one of my objections, but requires rates to have different units. We could allow that to be the mathematical definition, and not worry about common usage; but it doesn't quite stand up to the sorts of things a mathematician thinks about. If you have a "rate" of one ounce per pound, does that become a "ratio" of 1:16 when you change units to ounces per ounce? In my mind, a change of units should not change what the ratio is called; it's a ratio between two quantities, not between the numbers currently being used to represent them. If we use one ounce of pigment per pound of paint, is that a rate or a ratio? I might call it a rate when I'm mixing it, because I think of it as "per" or "for each," but a ratio when I'm using it, because it just describes the mixture!
The distinction can become very cloudy!
Finally, let's check a dictionary. According to Merriam-Webster, http://m-w.com/ we have "Ratio 1 a : the indicated quotient of two mathematical expressions b : the relationship in quantity, amount, or size between two or more things : PROPORTION" "Rate 3 a : a fixed ratio between two things b : a charge, payment, or price fixed according to a ratio, scale, or standard: as (1) : a charge per unit of a public-service commodity (2) : a charge per unit of freight or passenger service (3) : a unit charge or ratio used by a government for assessing property taxes (4) British : a local tax 4 a : a quantity, amount, or degree of something measured per unit of something else b : an amount of payment or charge based on another amount; specifically : the amount of premium per unit of insurance" Though this isn't necessarily a mathematician's or teacher's definition, it supports my sense that "ratio" is generic, while "rate" applies to several slightly different kinds of ratios. A rate generally involves a "something else," either two different kinds of units (such as distance per time), or just two distinct things measured with the same unit (such as interest money per loaned money). I don't know whether that helps, but at least it may let you move on to more significant issues.
My final statement here seems most reasonable. The answer is 
In the questions below, we will be implicitly restricted to ratios of the non-rate sort, ratios between either two quantities of the same type (e.g. pounds of butter and pounds of sugar) that could be combined into a single whole, or, even more so, that whole and a part of it. Rates will not fit any of this discussion.
Can a ratio be “part to whole”?
Next, from 2003:
Definition of Ratio I am a copy editor for a public-relations firm that works with clients in high technology. Recently, I lost a battle with other editors on the staff who insisted that a construction such as "eight out of ten" is a ratio. I said I didn't think it met the mathematical criteria for ratio, but since I don't know precisely how "ratio" is defined by mathematicians, I was unable to argue persuasively - and don't know for a fact that my co-editors aren't right, that "eight out of ten" (or 8 out of 10) IS a ratio. Can you help?
Suzanne presumably sees ratios as necessarily “part to part” rather than “part to whole” (like a fraction). Can a fraction be called a ratio?
I answered:
Hi, Suzanne. This is as much an English language question as a math question, and that makes it very confusing. Words like this are not used as consistently as you might expect, even among math teachers or mathematicians. (That's partly because mathematicians today don't tend to pay much attention to ratios, and therefore don't have to define them carefully.) My first impression is that we TEND to think of ratios as comparisons of, say, the number of boys to the number of girls, rather than of a part to a whole, but that the term "ratio" does not necessarily exclude the latter. So it might not be technically wrong to use it that way, but depending on the context there might be clearer ways to phrase what you want to say, so as to avoid suggesting that the comparison is part to part.
So, if you just mentioned a ratio, I would probably expect the ratio of two parts of a whole; but you might not mean that.
As in the question above, though, I like to confirm my opinion on questions like this:
Then I did a little searching. For word questions, I like to see what a dictionary says, since lexicographers know how to make distinctions between words (though they often don't understand the mathematical distinctions). Merriam-Webster (m-w.com) says "ratio 1 a : the indicated quotient of two mathematical expressions b : the relationship in quantity, amount, or size between two or more things : PROPORTION" This agrees with my general sense of the word: any quotient can be called a ratio, but in particular it tends to compare two distinct things. But, of course, we make a distinction between ratio and proportion, and they call them synonymous. How do they define the latter?
Here is another ambiguity: “Proportion” can be used either as a synonym for “ratio”, or as an equation of ratios:
"proportion 3 : the relation of one part to another or to the whole with respect to magnitude, quantity, or degree : RATIO 4 : SIZE, DIMENSION 5 : a statement of equality between two ratios in which the first of the four terms divided by the second equals the third divided by the fourth (as in 4/2=10/5)" Their definition 5 is the one we use technically, in distinction to ratio. But look at their definition 3: When they consider this synonymous with ratio, they specifically include relations of part to whole as well as part to part. That would say that your example IS a ratio.
So much for the dictionary …
How about math sites? Mathematicians, as I said, don't deal with this much, but math teachers do. Here is one reference I found: Ratio. Fraction. What's the Difference? http://www.sci.tamucc.edu/txcetp/cr/math/rf/RatioFraction.pdf "Due to common notation, students often improperly interchange the ideas of ratio and fraction. Through this lesson students will learn why the two are different ideas and when they actually can overlap. ... "Discuss the fact that fractions always illustrate a "part to whole" relationship while ratios can be used to illustrate a much larger set of relationships; such as part to part and whole to part." This seems to say that a fraction always refers to part of a whole, but a ratio can indicate a variety of relationships. There are some examples in a worksheet, but no answers.
This agrees with my impression.
The next page I found happened to be the answer sheet, and illustrates what they mean:
"2. Two out of every five students in this class plan to be middle school teachers.
Circle One: Ratio Fraction [Both]
"Reasoning:
"This is definitely a ratio of future middle school teachers to total students in the class of 2:5. In addition, since the ratio is part to whole, it can also be thought of as a fractional relationship. 2/5 of the students in the class plan to be middle school teachers."
So "two of every five" is considered a ratio.
I added a little more, and closed:
I found lots of pages that talk about ratio of part to whole, as well as part to part. So it looks like you've lost - but I'd still like to see the context in which you don't think the word "ratio" fits, because even if something CAN be called a ratio, there may be reasons not to use the word "ratio" in a particular sentence.
Suzanne responded:
Wow, thank you for the incredibly thorough explanation you so quickly provided. I admit to be being math-phobic, but I found your examples illuminating. Thanks again, and I'll quit arguing with my co-workers. Or find something else to argue about, more likely.
Can a fraction be part-to-part?
A question from 2013 questioned that:
Ratios and Fractions: A Reconciliation I am emailing you for support. Three math teachers were having a discussion today about how fractions show a part of a whole, but do not express part to part. Here is an example. In a class of 25 students, there are 15 boys and 10 girls. This represents the ratio of boys to girls: 15:10 But this cannot translate to 15/10. Saying 15 boys out of 10 girls would be like comparing apples to oranges! You can say 15/25 (15 boys out of 25 students) 10/25 (10 girls out of 25 students) One teacher offers this website below as her support that fractions can be "part to part," and that you can express a "part to part" as a fraction. https://www.khanacademy.org/math/pre-algebra/rates-and-ratios/ ratios_and_proportions/v/introduction-to-ratios-new-hd-version I disagree. But when I consult Dr. Math -- my "go to" site for math questions -- I find this explanation: http://mathforum.org/library/drmath/view/63884.html Can a fraction be part to part?
The Khan Academy link is dead, so we can’t be sure exactly what it said; I can’t find a current video there that shows such ratios as fractions.
That last reference is our previous question, where I, too, supported “part-to-whole” ratios. But is this the same as a fraction?
I answered:
Hi, Anne. The last page you referred to (by me) says essentially that a fraction is a ratio -- that is, a ratio can compare part to whole. You are looking at the opposite question: Can a fraction compare part to part? One thing that gets in the way of this sort of discussion is that in practice we find that we can WRITE most ratios as fractions, unifying the two concepts so that we don't need to learn two entirely separate concepts (as they did in the old days). So even though something is fundamentally a part-to-part ratio of 3:2, and not a fraction, we can WRITE it as 3/2 and do all the same simplifying we'd do with any fraction.
That is, it is common to write ratios using the slash (“/”); but does that mean such a ratio is a fraction?
There are major differences, of course -- a ratio can be 4:0 while a fraction can't be 4/0; you can have compound ratios like 2:3:4, which don't correspond to any sort of fraction; and you don't have "improper" ratios or "mixed" ratios. But we do often blur the distinction in cases where it doesn't affect how we work with a ratio. This, I think, is what Khan is doing: not saying that the ratios he's working with ARE fractions, but that we can use fraction NOTATION as a way to write and work with ratios. Anyone with experience in math will probably do just what he does -- develop a habit of writing ratios as fractions when it makes sense to do so -- because that simplifies our thinking.
Most of the work we do with ratios is identical to what we do with fractions, so we tend to borrow the notation. But not all ratios make sense that way.
Where the fraction notation becomes especially helpful is with algebra. In the old days, proportions had to be written using ratio notation (and couldn't even use "="):
3:2 :: x:10
To solve this, they would say "the product of the means [the middle two numbers] equals the product of the extremes [the outside numbers]," and write
2*x = 3*10
Today, we tend to write the ratios in a proportion as fractions, so that we can use algebra (and/or "cross multiplication") to solve:
3 x
--- = --- ---> 2x = 3*10
2 10
So, while there is value in teaching a distinction between ratios and fractions in terms of their applications and meanings, there is also value in learning to transform one into the other in order to work with them conveniently. An overly rigid distinction in the elementary grades might even get in the way of learning later on, and can at least lead to deprecating good techniques used by adults.
The old notation and terminology is discussed in Many Ways to Solve a Proportion.
Notice that in my example, the “fractions” are improper, but that doesn’t matter because we are just working with numbers, not with parts and wholes.
In fact, a ratio is closely related to a fraction even conceptually. If the ratio of boys to girls is 3:2, then the number of boys is 3/2 of the number of girls; there are 1 1/2 boys for every girl. So the boundary between ratios and fractions is not impermeable. What is my answer to your question, you ask? In my opinion, both sides in your disagreement are right: You're right that part-to-part ratios are not really fractions, but they're right that writing them as fractions can be appropriate.
Are all fractions ratios?
We’ll close with a 2014 question:
Fractions as Ratios: Wholly or Partially? How would ratio and fraction be placed in a Venn diagram? Would fraction fall totally within the ratio circle, or only partially within it? I guess another way to ask this question is, "Are ALL fractions ratios?" I have asked this question at the university level and there is disagreement. What is your opinion?
Which is right?
Or maybe this?
I answered:
Hi, Donna.
I would expect some differences of opinion, particularly because each word is used in various contexts, and one might give a different answer depending on what one is thinking about when the question is asked. It would be illuminating to hear what was said by the people you asked, not just as a true/false question, but in terms of the reasons they give.
I myself gave a generally affirmative answer to this question here:
Definition of Ratio
http://mathforum.org/library/drmath/view/63884.html
Here we're thinking of both ratios and fractions as representations of a relationship between numbers, where ratios can be "anything to anything," while fractions are always "part to whole."
This again is our second question above, where I seem to make fractions a subset of ratios.
But from a different perspective, I would consider a fraction to be an entirely different kind of thing from a ratio: a fraction is a way of writing a NUMBER -- namely as an "indicated quotient" -- whereas a ratio is not a number at all, but a comparison of two numbers. In this sense, we write fractions all the time without thinking at all of "part-to-whole" relationships or any such application of the concept. For example, when I write the equation 2/3 x - 1/2 = 5/6, I would not normally think of those fractions as ratios. On the other hand, you could choose to represent them as ratios if you had reason to.
You may notice that we quoted above a definition of a ratio as an “indicated quotient”! I didn’t fully approve of that; “quotient” means division, and a ratio is not actually a division, but a relationship. (But note that the colon we use in ratios is used in many countries as the division sign, so the distinction is even more confused.)
I wrote from this perspective here, emphasizing different senses in which the word "fraction" is used:
What Exactly is a Fraction?
http://mathforum.org/library/drmath/view/56034.html
So, as a relationship between numbers, fractions can be considered to be a subset of ratios, only written differently. But as a way of writing a number, a fraction is not even in the same universe as a ratio, so you wouldn't put them in the same Venn diagram.
That question is the basis of What is a Fraction, Really?
In summary, when asked specifically about the relationship between ratios and fractions, I would say the latter are a subset, and would make the Venn diagram; but when asked what a fraction IS, without reference to a Venn diagram, I might not mention ratios at all. And if you then asked me whether all fractions are ratios, I'd say, "Of course not." That may be what your respondents are doing -- but I'd still like to hear some of their explanations for their opinions, which might reveal even more perspectives I haven't thought of.
Putting it all together
Here is an attempt at a Venn diagram including all that we’ve said here:
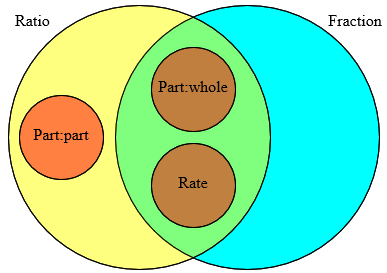
A part-to-part ratio can be represented as a fraction, but does not represent a fraction. When seen as a ratio at all, a fraction might represent a part-to-whole ratio, or a rate (which, again, involves different sorts of quantities that are not part of one whole).
If we think of fractions as numbers and not ratios, we get this alternative:

Now a fraction is just a way to write a number. We could say that a rate is actually a number, and so belongs on the right side; but I won’t try get everything exactly right!
