Having just discussed why we use compass and straightedge in geometry, let’s flip that around and look at a common question at the more elementary level: How do you use a ruler to measure or draw a line of a given length? The usual issue here is working with the fractional markings on an inch ruler, but we’ll also touch on issues with a metric ruler — and even how the ruler got its name. (It’s more interesting than you may think!)
Reading the marks
We can start with an early question and answer (1997) that we regularly used as a reference for students asking about this:
Reading a Ruler I need to read a ruler, and I can't. Can you help me?
Doctor Rob replied:
I hope so. There are two main kinds of rulers in general use, and other, more obscure kinds. We will ignore the obscure ones.
Here is an example of a ruler that combines the two kinds we’ll be seeing (inch on top, metric on the bottom):
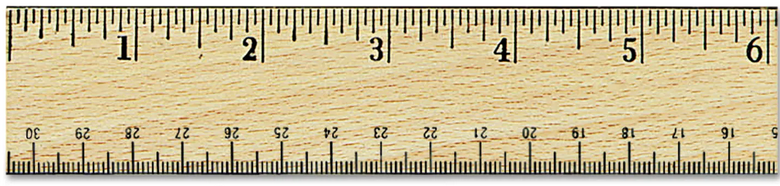
Fractional inch rulers
First there is the ruler marked in inches, and each inch is subdivided into 16 parts. The lines on it look something like this sketch of a part of a one-foot ruler: 8 9 | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | ----------------------------------- D C D B D C D A D C D B D C D The lines have different lengths to help you figure out what lengths they represent. The shortest lines (D) represent an odd number of sixteenths of an inch. The next shortest lines (C) represent an odd number of eighths of an inch. The next shortest lines (B) represent an odd number of quarters of an inch. The next shortest lines (A) represent an odd number of halves of an inch. The longest lines (8 or 9) represent whole inches, and are numbered. The lines labeled 8 and 9 above mark points on the edge of the ruler that are eight and nine inches from the left-hand end of the ruler. All distances are measured from that same left-hand end of the ruler, which could be, but probably isn't, marked "0".
Some rulers have an actual mark for 0, while others just start at the edge of the stick.
This traditional set of markings is based, not on 10 as in our decimal system of counting, but on 2: the denominator is always a power of 2. (As we’ll see below, the markings may go only to eighths, or go further than 16, but a denominator of 16 is common.) We’ll have different pictures below that may be easier to follow.
The line halfway between them labeled A above marks a point on the edge of the ruler, which is 8 1/2 inches from the end. That makes sense because 8 1/2 is halfway between 8 and 9.
The next shorter lines labeled B above are halfway between 8 and 8 1/2, and halfway between 8 1/2 and 9. The former one marks 8 1/4 inches, and the latter marks 8 3/4 inches. These make sense because 8 1/4 is halfway between 8 and 8 1/2, and 8 3/4 is halfway between 8 1/2 and 9. In the words of arithmetic,
8 + ([8+1/2]-8)*(1/2) = [8+1/4],
^^^^^^^^^
distance from 8 to [8+1/2]
and [8+1/2] + (9-[8+1/2])*(1/2) = [8+3/4].
^^^^^^^^^^^
distance from [8+1/2] to 9
Likewise, the next shorter lines labeled C above are halfway between 8 and [8+1/4], between [8+1/4] and [8+1/2], between [8+1/2] and [8+3/4], and between [8+3/4] and 9. They must therefore mark the distances [8+1/8], [8+3/8], [8+5/8], and [8+7/8], respectively.
Finally, the shortest lines labeled D above are halfway between adjacent pairs of longer lines, and mark [8+1/16], [8+3/16], ..., [8+15/16].
That looks as if there were a lot of arithmetic with fractions needed here; it will get simpler in a moment!
When I measure a distance, I put the "0" end of the ruler at one end, and then pick the mark on the ruler which is closest to the other end of the distance. The nearest inch line to the left gives me the number of whole inches. I then figure out whether this line is a 1/16 line (shortest), a 1/8 line, a 1/4 line, a 1/2 line, or an inch line. That tells me what the denominator of the fraction of an inch will be. From the inch line I count the lines the same length as my chosen one using odd number: "1, 3, 5, 7, ..." until I find my line. That tells me what the numerator of the fraction of an inch will be. I then combine the number of whole inches with the fraction to get the distance.
This is what replaces the arithmetic above! We count down in size to the type of mark we are looking at (doubling the denominator), then count odd numbers to find the numerator.
Metric rulers
The metric system uses decimal numbers rather than fractions, so here we use tens.
The other common kind of ruler measures centimeters instead of inches. Each centimeter is divided into 10 parts (each called a millimeter). The lines on it look something like this sketch of a part of such a ruler: 13 14 | | | | | ||||||||||| ------------- CAAAABAAAAC The longest lines labeled C represent whole centimeters. The next longest line labeled B represents a half centimeter. The shortest lines labeled A represent tenths of centimeters, or millimeters. Since 1/2 = 5/10, the B line also represents 5 millimeters.
The fractional ruler used twos because those are easy to count; here we have a special mark at 5 for the same reason. In effect, the markings use base 2 and 5 (like Roman numerals).
To measure a length, put the left end of the ruler, which could be labeled "0" but probably isn't, at one end, and pick the closest mark on the ruler to the other end. Find the closest centimeter mark to the left of your mark. That will tell you the number of whole centimeters (13 in the above example). The denominator of the fraction of a centimeter is fixed at 10. The numerator is found by counting from the whole centimeter mark you found above, and the medium-length lines B help you count by showing you where 5 tenths or half a centimeter is. If you are close to the "13" mark, you count up as you move to the right starting with "0" for the "13" mark itself. If you are close to the "B" mark, you can count up as you move to the right or down as you move to the left, starting with "5" for the B mark itself. If you are close to the "14" mark, you can count down as you move to the left, starting with "10" for the "14" mark itself. This will tell you the numerator of the fraction of a centimeter.
Normally we either write the length in millimeters, or use decimals rather than fractions. We’ll have an illustration of this later.
Naming the fractions
Our next answer is from 1999, and just fills in some details in the pictures:
Finding Fractions on a Ruler My question is simple. I want to know how to read all the measurements within 1 inch. We know that every line on a ruler or tape measure (whichever) has a fractional meaning. I want to learn how these fractions are arranged. I hope you understand what I mean by all fractions in an inch. Example: where is 7/8 of an inch compared to 5/16 of an inch on a tape measure? I know where the basic measurements are, such as 1/4, 1/2, and 3/4 of an inch. It's just the other measurements that I don't get.
I first referred to the answer above, and then added some more detailed diagrams:
I'll give you a simpler, quick answer that may meet your needs. Here's a ruler showing 16ths, with the meaning of each mark indicated: 0 1 | 1/2 | | | | | 1/4 | 3/4 | | | | | | | 1/8 | 3/8 | 5/8 | 7/8 | | | | | | | | | | | 1/16 | 3/16 | 5/16 | 7/16 | 9/16 | 11/16 | 13/16 | 15/16 | | | | | | | | | | | | | | | | | | +---+---+---+---+---+---+---+---+---+---+---+---+---+---+---+---+ Some rulers only go to eighths, and it might be easier to start with that: 0 1 | 1/2 | | | | | 1/4 | 3/4 | | | | | | | 1/8 | 3/8 | 5/8 | 7/8 | | | | | | | | | | +-------+-------+-------+-------+-------+-------+-------+-------+ 0 1/8 2/8 3/8 4/8 5/8 6/8 7/8 1 As the marks go down in size, the denominator of the fraction doubles. The biggest mark between two inches is the half; between that and either inch mark the next largest mark is the quarter inch; and so on. To find eighths, just go down the scale finding 1/2, then 1/4, then 1/8. All the marks that are that size or bigger are eighths: 1/8, 2/8 (which is the same as 1/4), 3/8, 4/8 (which is the same as 1/2), 5/8, and so on. If you want, you can just count all the marks that are the same size, counting by odd numbers: 1/8, 3/8, 5/8, 7/8.
As Doctor Rob explained, we can count only odd numbers; or we can just count all the lines of at least the length of the one we are reading. This is because all the even numbers correspond to fractions with a smaller denominator (and longer lines). For example, the 3/4 mark is the third line of at least its length, or we can count 1, 3 looking only at lines of the same length.
Try doing the same thing on the 16ths ruler above; the only hard part will be to ignore the smaller 16ths marks while you're looking for 8ths. To find 5/8, for example, find which size marks are 8ths, then count 1, 3, 5/8 until you find it. Now you should be able to work out 32nds yourself: 0 1 | 1/2 | /2 | | | | 1/4 | 3/4 | /4 | | | | | | 1/8 | 3/8 | 5/8 | 7/8 | /8 | | | | | | | | | | 1/16 | 3/16 | 5/16 | 7/16 | 9/16 | 11/16 | 13/16 | 15/16 | /16 | | | | | | | | | | | | | | | | | | 1 | 3 | 5 | 7 | 9 | 11| 13| 15| 17| 19| 21| 23| 25| 27| 29| 31| /32 | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | +-+-+-+-+-+-+-+-+-+-+-+-+-+-+-+-+-+-+-+-+-+-+-+-+-+-+-+-+-+-+-+-+
The 16th ruler would, of course, just lack the smallest lines here.
Measuring an object
In 2000, Doctor Ian answered a very similar question with details on how to find the length of an object. The question was this:
Reading a Ruler II What do the other little lines on the ruler stand for?
Doctor Ian first showed a metric ruler:
A metric ruler divides a meter into 100 centimeters,
0 1 2 99 100
|___|___|___ ... ___|___|
and divides each centimeter into millimeters,
0 1 2
| | |
|||||||||||||||||||||
^ ^
10 mm 19 mm
1 cm 1.9 cm
0.1 m 0.19 m
which means that the distance between any of the smallest lines is
1/1000 of a meter.
This version lacks the longer line at 5; but we can see here that we are counting millimeters (mm), which are tenths of a centimeter, so that 19 mm is the same as 1.9 cm.
After showing fractional rulers as we’ve seen above, he took it further, showing exactly how to read a length:
So let's say I want to measure something with a ruler. I set it down
next to the ruler,
0 1 2 3
| | | |
| | | | | | |
| | | | | | | | | | | | |
|_|_|_|_|_|_|_|_|_|_|_|_|_|_|_|_|_|_|_|_|_|_|_|_|
############################################
############################################
############################################
############################################
^_______________^ 2 to 3 in
^_______^ 2-1/2 to 3 in
^___^ 2-1/2 to 2-3/4 in
^_^ 2-5/8 to 2-3/4 in
and I find the two closest inch-marks that are on either side of it. In the picture above, these are the 2- and 3-inch marks. So we know that the thing is between 2 and 3 inches long.
If all we care about is the nearest inch, we’re done! It’s between 2 and 3 inches, and closer to 3. But we want to be as precise as possible:
Next, we look at the 2-1/2 inch mark. The thing extends past it, so we know that the thing is between 2-1/2 and 3 inches long.
Next, we look at the mark halfway between those, the 2-3/4 inch mark. The thing doesn't extend quite that far, so we know that the thing is between 2-1/2 and 2-3/4 inches long.
And so on, until we've identified the two closest marks that are on either side of the end of the thing we're measuring. At this point, if it's very close to either mark, we can just call that the measurement. Or if it's right in between, we can take the average. In the example above, that would be:
5/8 + 3/4
2 and ----------- inches
2
5/8 + 6/8
= 2 and ----------- inches
2
11/8
= 2 and ----------- inches
2
= 2 and 11/16 inches
Note that you can measure something to the nearest 16th of an inch, even though the ruler is only marked to the nearest 8th of an inch.
An alternative way to do this last calculation is just to see that it’s about 1/16 more than 5/8, which is 10/16, so we estimate it as 11/16.
Can you just count lines?
The next question, in 2005, called for a still more detailed explanation:
Reading a Ruler III My daughter came home with a worksheet that had a ruler on it and it has letters on the ruler in which she is supposed to say the place (value) the letter represents on the ruler. Now it's been a while since I have done this. I am looking for simple, basic instructions to teach a child how to read a ruler. Of course I can instantly tell you where a 1/2 is; however, the rest is very vague to me. I somehow remember that we used to count the lines--where the line falls on the ruler is the top number of the fraction and how many lines in between 0 to 1 inch represents the bottom number (the whole). Is this correct? I like your examples on how to read a ruler. However, if you can just count where the line is and put that in the numerator and count how many lines make the whole and put that in the denominator that would be helpful to make it so that everyone truly understands how to read the ruler.
Doctor Ian assumed that Mary had read the answers above, and needed more:
Let's look at an example. Suppose I'm measuring something that's something and 7/16 of an inch long.
| |
| | |
| | | | |
| | | | | | | | |
| | | | | | | | | | | | | | | | |
-----------------------------------
xxxxxxxxxxxxxxxx
Here we see just the last inch of the object; the “something” would be the label on the inch mark at the left.
In practice, I'd look at the 1/2 mark, and say "That's too big." So I'd look at the 1/4 mark, and say "That's too small." So now I know it's between 1/4 and 1/2, i.e., between 1/4 and 2/4, which is between 2/8 and 4/8. What's halfway between them? 3/8. So I look at 3/8, and say "That's too small." So now I know it's halfway between 3/8 and 1/2, i.e., between 3/8 and 4/8, which is between 6/16 and 8/16. What's halfway between them? 7/16. And that works. So I'm done.
Another way would be to just see that it is one small mark (1/16) less than 1/2, and subtract; but that requires work with fractions. We’ll get to the “just count” approach Mary wants soon!
The whole system is based on successively breaking things into halves, so I just go with the flow. That is, you can imagine a ruler where the markings only go down to 1/2 an inch: | | | | | | | | | | | | | | ----------------------------------- 0 1/2 2/2 = 1 If we decide that's not fine enough, we double the denominators, | | | | | | | | | | | | | | ----------------------------------- 0 2/4 4/4 = 1 and that gives us room for more numerators: | | | | | | | | | | | | | | | | | | | | ----------------------------------- 0 1/4 2/4 3/4 4/4 And so on, through 8ths and 16ths and even 32nds, if we can make our markings finely enough. Of course, this is all but impossible to deal with unless you're pretty facile at converting between equivalent fractions with powers of 2 in the denominator, e.g., 1/2 = 2/4 = 4/8 = 8/16 = 16/32 That's the basic skill you need to make the system work.
What you don’t do this often enough to develop that skill?
The idea of counting all the marks to figure out what the denominator should be would work in theory, but in practice it would be pretty slow. But with some practice, you could do something sort of equivalent but quicker.
That is, you could start by identifying what size mark is next to the end of the thing you're measuring:
| |
| | |
| | | | |
| | | | | | | | |
| | | | | | | | | | | | | | | | |
-----------------------------------
xxxxxxxxxxxxx^
|_________ This is the size I want.
Next, you could look at the 1/2 mark, and back up halfway to the 1/4 mark. If that's the right size, you can now just count quarters. If it's still too small, back up halfway to the 1/8 mark. If that's the right size (in the example above, it is), you can just count eighths. And so on.
Note that when you're 'counting quarters' or 'counting eighths', you have to count any marks that are at least as large as the one you're using; but you can ignore any that are smaller. So that's pretty straightforward, but again it require at least a little facility with powers of 2 in the denominator.
In this example, we’ve identified the mark as an eighth, so we can just count marks this big or longer: 1, 2, 3; or, as we’ve said above, just count the actual eighth marks by twos: 1, 3.
Why is it called a ruler? Isn’t that a king?
Several of us are interested not only in numbers, but also in words. I find the following fascinating:
History of the Word I was just wondering, why is a ruler called a ruler?
Doctor Rick answered:
Do you sometimes look at a word you see every day, and suddenly it seems strange? I do that. I, too, wonder how a word can mean two things that seem very different. The history of words (called etymology) has lots of strange stories that sort of make sense when you think about them. A good place to start answering questions about words is a dictionary. Looking in my dictionary (Random House Webster's College Dictionary), the etymology listing under "rule" says this: [1175-1225; (n.) ME riule, reule < OF riule < L regula straight stick, pattern, der. of regere to fix the line of, direct (see -ULE). In other words: The word was first used around 1200. Before that (in "Middle English", it was spelled with an "i" or an "e". Why? Because it was spelled that way in Old French. The French took the Latin word "regula", dropped the g and changed the a to a silent e. (The French did that sort of thing a lot, dropping letters and not pronouncing a lot of the letters they kept!)
English does that too …
The Latin word "regula" meant a straight stick. That's a ruler: a device for making straight lines. Whether the Romans put marks on their rulers to measure lengths, I don't know, but apparently the most important thing about it was that it was straight. Why do I say that? Because it comes from the word regere, which meant "to guide or direct, to make straight." When you use a ruler to make a line, it keeps the line from going astray in either direction; it guides your pencil the way you want it to go.
We still (sometimes) talk about “ruled” paper, meaning it has straight lines drawn on it.
Now that we know that the word from which we get "rule" or "ruler" had "a straight stick" as its primary meaning, our question gets turned around: How did "rule", meaning "a straight stick", come to mean "tell others what to do", or "a law that we have to follow"? But maybe you can already see the answer. A ruler sets the standard; it's a pattern to be followed. If you put a ruler next to a line, you can tell right away if the line is crooked. A rule or regulation (notice where *this* word comes from?) sets a standard, so everyone knows if you "break the rule". Someone who sets the rules for others to follow is called a ruler.
Did you notice that the original meaning of ruler was just “straightedge”, without reference to having marks on it?
There are lots of other words related to rule, ruler and regulation. Something that is "regular" follows the rules; something irregular breaks the rules. The Romans put the prefix de- in front of regere and it became derigere: to straighten or direct--we get the word direct (and the word dirigible, something that can be steered) from derigere. And notice the "rect" part of direct: all sorts of words, such as rectangle, and even right (angle), come from Latin rectus, which means "made straight" from regere, to make straight. There's more on that here: Left Angles http://mathforum.org/library/drmath/view/58420.html If you found that interesting, I hope you can take a Latin class in a few years. You'll learn a lot about words that way. If you lost interest after the first paragraph, ... anyway, I'm done now.
Next time, let’s look at protractors!

Pingback: Using a Protractor – The Math Doctors